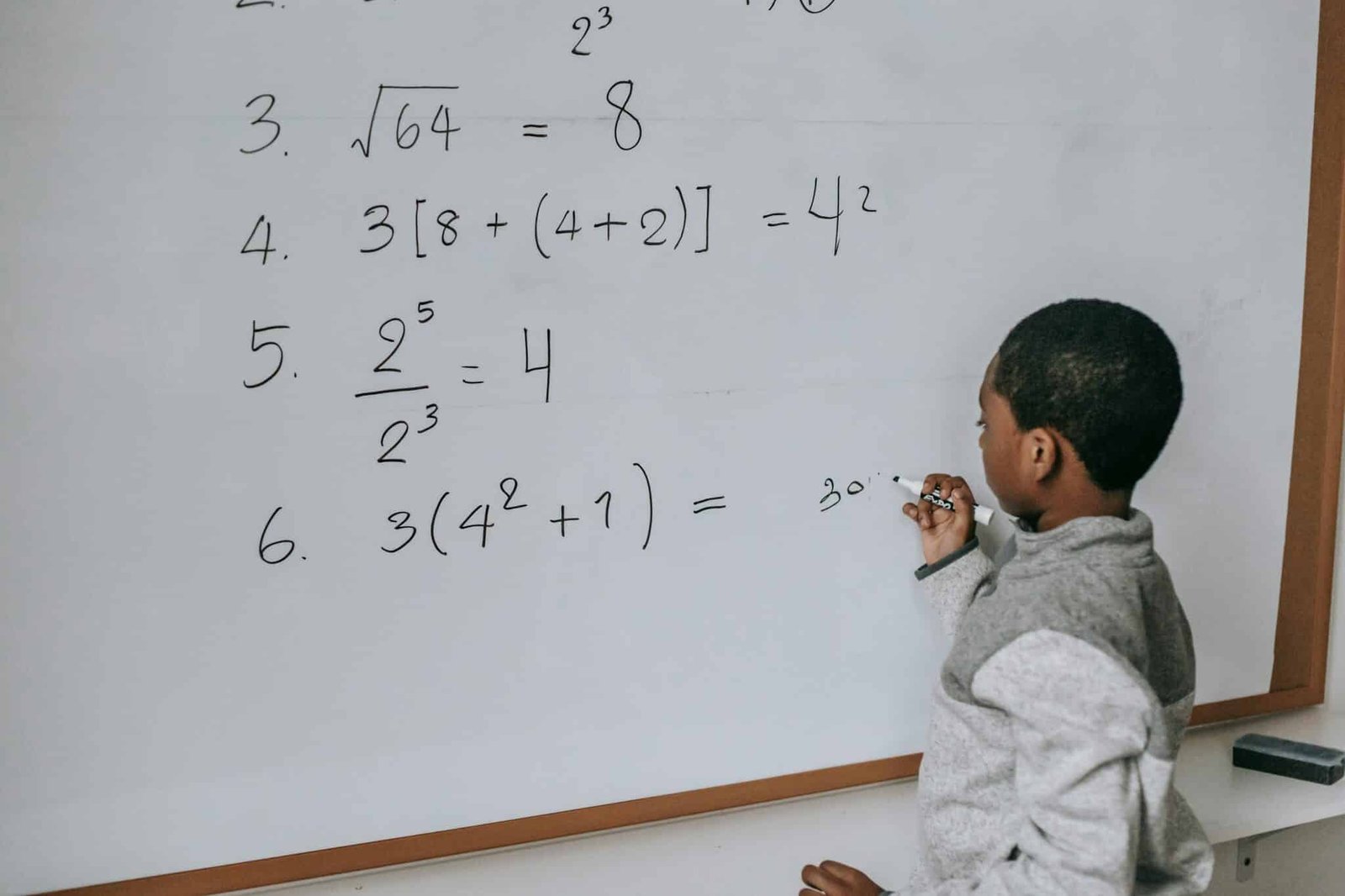Square root equation examples help students understand mathematical concepts because of easy solutions. The examples clarify complex problems. It makes them easier to solve. Additionally, they highlight common mistakes, further improving accuracy. You can analyze different approaches to develop problem-solving skills. Observing textbook examples enhances confidence. The students apply square root equations correctly in academic and real-world scenarios.
Why must you observe square root equation examples in the textbook?
Understanding square root equation examples
Examining square root equation examples in textbooks helps students build a solid foundation. As a result, you can clear explanations break down complex problems. They make abstract concepts easier to understand. When you follow structured steps, learners develop logical thinking. Additionally, exposure to different approaches improves adaptability. The students can handle various problem types effectively.
Textbook examples offer valuable insights. They demonstrate correct methodologies. Through repeated exposure, students become familiar with solving strategies. It reduces confusion. Moreover, seeing multiple examples reinforces key concepts to make problem-solving smoother. Understanding math algebra means you can apply the knowledge confidently in different mathematical scenarios.
square root equation examples Enhance Accuracy
Secondly, you can carefully analyze square root equation examples to minimize common calculation errors. The solutions help students recognize patterns to improve accuracy. Additionally, observing detailed explanations clarifies misconceptions to ensure the correct application of rules. As students refine their approach, they solve problems more efficiently. Strong attention to accuracy significantly enhances performance in both practice and exams.
You can exposure to correctly solved equations strengthens problem-solving techniques. Seeing multiple examples allows students to compare methods. It reinforces understanding. Furthermore, consistent practice reduces reliance on guesswork. Moreover, it leads to greater confidence. Mastering square root equations through textbook examples. They ensure students apply their knowledge precisely in academic and real-world situations.

Building Problem-Solving Confidence
Thirdly, you can follow the square root equation examples to build confidence. You can assume the examples are a genre to help students understand how to approach different equations. Additionally, structured examples illustrate multiple methods to help learners choose the most effective strategy. As students practice more, their ability to solve equations independently increases significantly over time.
Confidence grows when students solve math word problems accurately. Textbook examples often include real-world problems to make them more engaging. Additionally, solving the examples builds familiarity with mathematical processes and reduces anxiety. Mastering square root equations empowers students to tackle more advanced topics with a strong sense of competence.
Supporting Independent Learning
Furthermore, using square root equation examples as study tools encourages independent learning. You can review the solutions to participate in the equation actively. Additionally, they can analyze different techniques so you can discover effective methods. Independent learning fosters critical thinking and allows students to approach mathematical challenges. You benefit from greater confidence and problem-solving efficiency.
Observing multiple examples helps students recognize patterns and apply concepts to new problems. As they progress, their ability to solve equations without assistance improves. Furthermore, textbooks provide structured guidance so that learners can assess their understanding. Developing independent learning skills prepares students for advanced mathematics and lifelong problem-solving challenges.
Increasing Retention and Application
Lastly, studying square root equation examples improves knowledge retention. It reinforces key concepts. Repeated exposure to solved equations strengthens memory and makes it easier to recall during exams. Additionally, seeing different problem variations enhances adaptability. It helps students tackle new questions effectively. A strong grasp of square root equations allows learners to apply their skills across subjects.
You can apply square root concepts in real-world scenarios to deepen understanding. Textbooks often provide practical examples. It shows how the equations relate to physics, engineering, and finance. The students recognize the connections to see the relevance of mathematics beyond the classroom. Strong retention and application skills enhance academic performance and future problem-solving capabilities.
Square root equation examples 1
Learn how to simplify the equation of √ (√9−√8). To solve this math, first we need to square 9. Then, we will get 3 plus square 8. Next, we need to write 3 as 2+1. It will help us to get the solution more efficiently. All Square root equation examples are tricky. However, you can solve the math with practice. Now, let’s see the guide.
√ (√9−√8)
Next, √ (√9−√4*2)
√ (√2+1−2√2)
Now, we apply a2 – 2ab + b2. The expansive formula is similar to (a+b)2
√ ((√2)2− 2√2√1 + (√1)2)
√ (√2 – √1)2
√2 – √1
Square root equation examples 2
This simplification question is very popular in admission, the math Olympiad, the GRE, and other lots of exams. The solution to √ (9^3+9^3+9^3) presents a systematic approach, inviting contestants to navigate through the complexities of exponents and radicals with precision and clarity.
Recognize the Pattern
First, observe that each term in the expression is 93. Since there are three identical terms, you can factor out 93. Therefore, recognizing the pattern simplifies calculations. In other words, you do not need to expand each power. Instead, you can separately compute each power. Furthermore, express the sum as 93 + 93 + 93. Next, we prepare to simplify.
Combine the Terms
Secondly, we will factor out the common exponentiated base. Initially, the example has three identical terms exist. Therefore, we can rewrite the sum as 3(93). Moreover, the step consolidates the expression and makes it easier to evaluate. In contrast, we will not add separately. Also, we will not multiply because it will simplify calculations. In short, we will group identical terms. As a result, you reduce computational complexity while ensuring accuracy.
Evaluate the Exponent
Thirdly, we will evaluate 93. Since 9=32, as a result, we can rewrite 93 as (32)3 to help simplify. As a result, we will multiply exponents to form (32)3=36. Thus, the approach streamlines calculations. At the same time, it maintains accuracy in addition to logical flow toward the next step.
Combine the Terms
As a result, the new translation is 36.3. The transformation simplifies the original problem into a straightforward multiplication. Moreover, you can rewrite the expressions into simpler components allows for easier computations. The strategic approach ensures an efficient calculation process. At the same time, you do not disturb the equation. It maintains numerical integrity. Now, we evaluate the 36.3 to proceed.
Breaking up the nth root
Now, we will break up the √36.3 into √36. √3. The approach simplifies the expression. Meanwhile, the equation maintains accuracy. After you have broken up the equation, you can analyze each component individually. As a result, the calculations are easier and more precise.
Take the Square Root
Subsequently, the new expression becomes (36)1/2.31/2. As a result, you can further simply the (36)1/2.31/2 to 33.31/2.
Final Answer
Thus, the final answer is 27√3. After you follow the structured steps, you can perform factoring, exponentiation, and multiplication, in addition to square root extraction. Thus, you ensure accuracy. Therefore, you can recognize patterns and simplify expressions. As a result, you have made calculations efficient. The systematic approach reduces complexity while maintaining precision. Therefore, 27√3 is the correct solution.
Study Square root equation examples
Square root equation examples enhance comprehension using step-by-step solutions. Therefore, you must study these examples to recognize patterns, avoid mistakes, and, also, build confidence. Additionally, repeated exposure strengthens retention and improves adaptability. Master the equations with essential skills for tackling advanced mathematical concepts. As a result, you apply logical reasoning in real-world situations.